retour

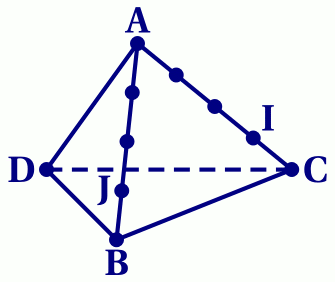
Dans le tétraèdre ABCD représenté ci-dessous, on a placé les points I et J tels que \[\overrightarrow{\mathrm{AI}} = \dfrac 3 4 \overrightarrow{\mathrm{AC}} \quad\text{et}\quad \overrightarrow{\mathrm{AJ}} = \dfrac 3 4 \overrightarrow{\mathrm{AB}} \]

1. Montrer que $\overrightarrow{\mathrm{JI}} = \dfrac 3 4 \overrightarrow{\mathrm{BC}}$.
   Corrigé
   D'après la relation de Chasles: \begin{align*} \overrightarrow{\mathrm{JI}} =&\overrightarrow{\mathrm{JA}}+\overrightarrow{\mathrm{AI}} =\frac 3 4\overrightarrow{\mathrm{BA}} + \frac 3 4\overrightarrow{\mathrm{AC}} =\frac 3 4\left(\overrightarrow{\mathrm{BA}}+\overrightarrow{\mathrm{AC}}\right)& \\ =&\frac 3 4\overrightarrow{\mathrm{BC}}.& \end{align*}
2. Que peut on en déduire pour la droite $(\mathrm{IJ})$ et le plan $(\mathrm{BCD})$?
   Corrigé
   Puisque $\overrightarrow{\mathrm{JI}}$ et $\overrightarrow{\mathrm{BC}}$ sont colinéaires, les droites $(\mathrm{IJ})$ et $(\mathrm{BC})$ sont parallèles.
   La droite $(\mathrm{BC})$ étant incluse dans le plan $(\mathrm{BCD})$, la droite $(\mathrm{IJ})$ est donc parallèle au plan $(\mathrm{BCD})$.
3. On considère les points E et F tels que \[\overrightarrow{\mathrm{AE}}=\overrightarrow{\mathrm{BC}} \quad\text{et}\quad \overrightarrow{\mathrm{AF}}=\overrightarrow{\mathrm{BD}}.\]
   1. $\left(\overrightarrow{\mathrm{BC}},\overrightarrow{\mathrm{BD}}\right)$ est-elle une base du plan $(\mathrm{BCD})$?
      Corrigé
      Les vecteurs $\overrightarrow{\mathrm{BC}}$ et $\overrightarrow{\mathrm{BD}}$ dirigent les droites sécantes $(\mathrm{BD})$ et $(\mathrm{BC})$; ils ne sont donc pas colinéaires et forment bien une base du plan $(\mathrm{BCD})$.
   2. Que peut-on dire des plans $(\mathrm{AEF})$ et $(\mathrm{BCD})$?
      Corrigé
      $(\overrightarrow{\mathrm{AE}},\overrightarrow{\mathrm{AF}})=(\overrightarrow{\mathrm{BC}},\overrightarrow{\mathrm{BD}})$ est une base du plan $(\mathrm{AEF})$ mais aussi du plan $(\mathrm{BCD})$. Ces deux plans sont donc parallèles.

      

retour

code : 776