EX-04-S05
retour
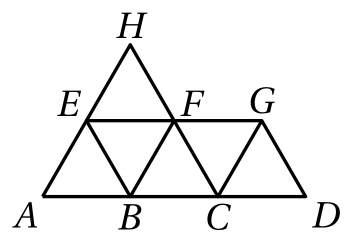
On considère la figure ci-dessous dans laquelle les triangles tracés sont équilatéraux.
-
Recopier et compléter les égalités suivantes avec des points de la figure.
-
$\overrightarrow{AB}+\overrightarrow{BE} = \overrightarrow{A\cdots}$;
Corrigé$\overrightarrow{AB}+\overrightarrow{BE} = \overrightarrow{\mathbf AE}$. -
$\overrightarrow{AB}-\overrightarrow{FB} = \overrightarrow{A\cdots}$;
Corrigé$\overrightarrow{AB}-\overrightarrow{FB} = \overrightarrow{AB}+\overrightarrow{BF} = \overrightarrow{\mathbf AF}$. -
$\overrightarrow{HE}+\overrightarrow{DG} = \overrightarrow{F\cdots}$;
Corrigé$\overrightarrow{HE}+\overrightarrow{DG} = \overrightarrow{FB}+\overrightarrow{BE} =\overrightarrow{\mathbf FE}$. -
$\overrightarrow{FC} - \overrightarrow{CB} = \overrightarrow{F\cdots}$.
Corrigé$\overrightarrow{FC} - \overrightarrow{CB} = \overrightarrow{FC}+\overrightarrow{CD} = \overrightarrow{\mathbf FD}$.
-
$\overrightarrow{AB}+\overrightarrow{BE} = \overrightarrow{A\cdots}$;
-
Remplacer les sommes vectorielles suivantes par un vecteur unique.
-
$\overrightarrow{CE}+\overrightarrow{AC}$;
Corrigé$\overrightarrow{CE}+\overrightarrow{AC} = \overrightarrow{AC}+\overrightarrow{CE} = \overrightarrow{AE}$ -
$\overrightarrow{EF}-\overrightarrow{FC}$;
Corrigé$\overrightarrow{EF}-\overrightarrow{FC} =\overrightarrow{EF}+\overrightarrow{FH} =\overrightarrow{EH}$
(ou encore $\overrightarrow{AE}$, $\overrightarrow{BF}$, $\overrightarrow{CG}$). -
$-\overrightarrow{BA} + \overrightarrow{AC}$;
Corrigé$-\overrightarrow{BA} + \overrightarrow{AC} =\overrightarrow{AB}+\overrightarrow{BD} = \overrightarrow{AD}$. -
$\overrightarrow{AB}+\overrightarrow{AE}+\overrightarrow{BC}$.
Corrigé$\overrightarrow{AB}+\overrightarrow{AE}+\overrightarrow{BC} =\overrightarrow{AB}+\overrightarrow{BF}+\overrightarrow{FG} = \overrightarrow{AG}$
-
$\overrightarrow{CE}+\overrightarrow{AC}$;
retour
code : 403